I. E. Irodov
First law of Thermodynamics, Heat Capacity
Solutions
----------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------------
Friends do not get surprise by looking the heading of today's post. The story behind today's post started in the year 2002 when I was preparing for IIT-JEE examinations. In those days it was a trend that if you can solve I. E. Irodov then you can crack JEE, but because of lack of knowledge and very short duration of preparation in which I have to finish my text books also I failed in solving Irodov. But today I am in a situation to solve it and I strongly recommend this book for all those student out there who are preparing for GATE and ESE. For mechanical engineering students I strongly recommend following sections of this book:
1.1 Kinematics
1.2 Fundamental equations of Dynamics
1.3 Laws of Conservation of Energy, Momentum, and Angular Momentum
1.5 Dynamics of a Solid Body
1.6 Elastic Deformations of a Solid Body
1.7 Hydrodynamics
2.2 The First Law of Thermodynamics. Heat Capacity
2.4 The Second Law of Thermodynamics. Entropy
2.5 Liquids. Capillary Effects
2.6 Phase Transformations
2.7 Transport Phenomena
Dear friends in this post I will be solving section 2.2 and in later post I will be solving Section 2.4 as they are the topics of this blog. the solutions of section 1.7 I have already uploaded in Slideshare and the link is there in one of the pages in my blog.
Point to be noted here is that I have solved the problems in mass bases not mole basis as well I have solved the problems as if they are derivation and if you want to arrive at the final answer which is given in book so please substitute the values and you will get the answer. There are few problems whose ans. I have not given I will be providing them in few days, so stay connected.
I have converted the problems in mass basis and in the form of derivative problem so they are different in form but same in concept with the problems in books
Let us start the solving the problems:
26. Demonstrate that
the interval energy U of the air in a room is independent of temperature
provided the outside pressure p is constant. Calculate U, if p
is equal to the normal atmospheric pressure and the room's volume is equal
to V = 40 m3.
28. Two thermally
insulated vessels 1 and 2 are filled with air and connected by a
short tube equipped with a valve. The volumes of the vessels, the pressures and
temperatures of air in them are known (V1, p1, T1
a nd V2, p2, T2). Find the air
temperature and pressure established after the opening of the valve.
29. Gaseous hydrogen
contained initially under standard conditions in a sealed vessel of volume V
was cooled by ΔT . Find how much the internal energy of the gas
will change and what amount of heat will be lost by the gas.
30. What amount of
heat is to be transferred to nitrogen in the isobaric heating process for that
gas to perform the work W ?
31. As a result of
the isobaric heating by ΔT m kg of a certain ideal gas obtains an amount of
heat Q . Find the work performed by the gas, the increment of its
internal energy, and the value of γ = Cp/Cv.
32. m kg of a certain
ideal gas at a temperature To were cooled isochorically so that the gas
pressure reduced n times. Then, as a result of the isobaric process, the gas
expanded till its temperature got back to the initial value. Find the total
amount of heat absorbed by the gas in this process.
35. m kilogram of a
certain ideal gas is contained under a weightless piston of a vertical cylinder
at a temperature T. The space over the piston opens into the atmosphere.
What work has to be performed in order to increase isothermally the gas volume
under the piston it times by slowly raising the piston? The friction of the
piston against the cylinder walls is negligibly small.
37. m kilogram of an ideal gas being initially at a temperature To were isothermally expanded n times its initial volume and then isochorically heated so that the pressure in the final state became equal to that in the initial state. The total amount of heat transferred to the gas during the process equals Q . Find the ratio γ = Cp/Cv for this gas.
38. Draw the approximate plots of isochoric, isobaric, isothermal, and adiabatic processes for the case of an ideal gas, using the following variables:
(a) p, T; (b) V, T.
39. One
kilogram of oxygen being initially at a temperature To is adiabatically compressed to increase its
pressure η times. Find:
(a)
the gas temperature after the compression;
(b)
the work that has been performed on the gas.
40. A certain mass
of nitrogen was compressed η times (in
terms of volume), first adiabatically, and then isothermally. In both cases the
initial state of the gas was the same. Find the ratio of the respective works
expended in each compression.
43. The volume of m
kilogram of an ideal gas with the adiabatic exponent γ is varied according to
the law V = a / T, where a is a constant. Find the amount of heat
obtained by the gas in this process if the gas temperature increased by ΔT.
44. Demonstrate that
the process in which the work performed by an ideal gas is proportional to the
corresponding increment of its internal energy is described by the equation pVn
= const, where n is a constant.
45. Find the heat
capacity of an ideal gas in a polytropic process pVn = const
if the adiabatic exponent of the gas is equal to γ. At what values of the
polytropic constant n will the heat capacity of the gas be negative?
46. In a certain
polytropic process the volume of argon was increased α times. Simultaneously,
the pressure decreased β times. Find the heat capacity of argon in this
process, assuming the gas to be ideal.
47.
m
kilogram of argon is expanded polytropically, the polytropic constant being n .
In the process, the gas temperature changes by ΔT . Find:
(a)
the amount of heat obtained by the gas;
(b) the work performed by the gas
48.
An
ideal gas whose adiabatic exponent equals γ is expanded so that the amount of
heat transferred to the gas is equal to the decrease of its internal energy.
Find:
(a)
the heat capacity of the gas in this process;
(b)
the equation of the process in the variables T, V;
(c)
the work performed by one mole of the gas when its volume increases η times if
the initial temperature of the gas is To.
49. m
kilogram of an ideal gas whose adiabatic exponent equals γ undergoes a process
in which the gas pressure relates to the temperature as p = aTα, where
a and α are constants.
Find:
(a)
the work performed by the gas if its temperature gets an increment ΔT;
(b)
the heat capacity of the gas in this process; at what value of α will the heat
capacity be negative?
50. An
ideal gas with the adiabatic exponent γ undergoes a process in which its
internal energy relates to the volume as U=aVα, where
a and α are constants. Find:
(a) the work performed by the gas and
the amount of heat to be transferred to this gas to increase its internal
energy by ΔU;
(b) the heat capacity
of the gas in this process.
51.
An ideal gas has a heat capacity Cv
at constant volume. Find the molar heat capacity of this gas as a function of
its volume V, if the gas undergoes the following process:
(a) T=T0 eαV (b)P=P0 eαV, where
Po, To, and α are constants.
52.
m
kilogram of an ideal gas whose adiabatic exponent equals γ undergoes a process p
= po + a /V, w here Po and a are positive constants. Find:
(a)
heat capacity of the gas as a function of its volume;
(b)
the internal energy increment of the gas, the work performed by it, and the
amount of heat transferred to the gas, if its volume increased from V1
to V2.
53.
m
kg of an ideal gas with heat capacity at
constant pressure Cp undergoes the process T = To +α V, where T o and
α are constants. Find:
(a)
heat capacity of the gas as a function of its volume;
(b)
the amount of heat transferred to the gas, if its volume increased from V1
to V2.
54.
For
the case of an ideal gas find the equation of the process (in the variables T,
V) in which the heat capacity varies as:
(a)
C = Cv + αT; (b) C = Cv + βV; (c) C = Cv + ap,
where
α, β, and a are constants.
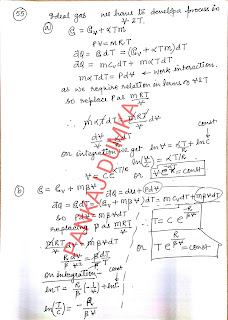
55.
An
ideal gas has an adiabatic exponent γ. In some process its heat capacity varies
as C = α/T, where a is a constant. Find:
(a)
the work performed by one mole of the gas during its heating from the
temperature To to the temperature η times higher;
(b)
the equation of the process in the variables p, V.
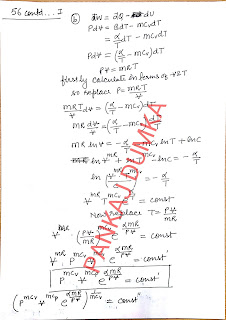
56. Find the work
performed by one mole of a Van der Waals gas during its isothermal expansion
from the volume V1 to V2 at a temperature T.
57.
m
kilogram of oxygen is expanded from a volume V1 to V2 at a constant temperature T .
Calculate:
(a)
the increment of the internal energy of the gas:
(b) the amount of the absorbed heat.
The
gas is assumed to be a Van der Waals gas.
58.
For
a Van der Waals gas find:
(a)
the equation of the adiabatic curve in the variables T, V;
(b)
the difference of the heat capacities Cp, — Cv as a function
of
T and V.
59. Two thermally
insulated vessels are interconnected by a tube equipped with a valve. One
vessel of volume V1 contains m
of carbon dioxide. The other vessel of
volume V 2 is evacuated. The valve having been opened, the
gas adiabatically expanded. Assuming the gas to obey the Van der Waals
equation, find its temperature change accompanying the expansion.
60. What amount of heat
has to be transferred to m Kg of carbon dioxide to keep its temperature
constant while it expands into vacuum from the volume V1 to V 2 ? The gas is assumed
to be a Van der Waals gas.
61. What amount of
heat has to be transferred to m kg of carbon dioxide to keep its temperature
constant while it expands into vacuum from the volume V1 to V2?
The gas is assumed to be a Van der Waals gas.
One more thing I want to say ( which I say always to every student) that please do not follow the solutions blindly. Firstly try yourself and if find difficulty in solving, then only look the solutions.
After few days I will be publishing solutions of next section (i.e. 2.4 --The Second Law of Thermodynamics. Entropy)
your comments are valuable to me, so please do write them. If any problem in any solution then also let me know.
Thankyou
All the Best.